A Level Maths: three things to consider for detailed reasoning
20 November 2024
Steven Walker, Maths Subject Advisor

Our A Level Maths and Further Maths specifications contain a list of command words along with their respective definitions. These are used throughout the question papers to help signpost to students what is expected in their written responses when answering questions.
In this blog, we will look more closely at the bold statement, ‘In this question you must show detailed reasoning’ (DR).
The specification states:
‘When a question includes this instruction learners must give a solution which leads to a conclusion showing a detailed and complete analytical method. Their solution should contain sufficient detail to allow the line of their argument to be followed. This is not a restriction on a learner’s use of a calculator when tackling the question, e.g. for checking an answer or evaluating a function at a given point, but it is a restriction on what will be accepted as evidence of a complete method.’
Demonstrate an understanding of the maths
There is no prohibition on the use of calculators, but to assess the mathematical understanding of certain content items we may use the DR to indicate where you must write down the mathematical steps that the calculator could have done.

Question 3 H640/02 2023
The calculator will change this numerical expression involving indices directly into a fraction with no mathematical knowledge required. This question requires students to demonstrate that they understand the reciprocal function, the cubic function and the square root function. The three marks represent the three steps that need to be seen.

Mark Scheme Q3 H640/02 2023
When answering DR questions you need to show enough working to demonstrate your understanding of the mathematics behind what the calculator can do.
Meet assessment criteria
There is a requirement in the DfE criteria to assess the ability to reason, interpret and communicate mathematically. The DR instruction can be used to emphasise that a question is assessing this objective.

Q3b H640/01 2022
Students should recognise that the DR here is looking for clear algebraic steps, and the ‘all points of intersection’ means that coordinate pairs are needed.
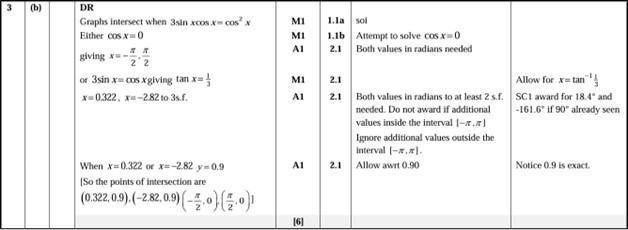
Mark Scheme Q3b H640/01 2022
A substantial number of students missed the cos x = 0 roots, which may have been avoided with a quick supporting sketch or making clear the implication of dividing by cos x.
Make sure you justify each line of working. Before dividing by f(x), check that f(x) = 0 is not a valid solution.
Support problem solving
Additionally, the DR statement may be used to remind students that showing their working is good mathematical practice. There is always a risk with longer, multi-step questions, especially those involving AO3 problem solving, that a student will try some different approaches on their calculator but then put little or nothing in writing that could be awarded marks.
The DR instruction helps to remind students that we can’t mark what we can’t see, so they must write down their working. It is also important to cross out anything known to be a mistake because credit can only be given for results obtained without wrong working (www).

Question 5(a) H240/02 2023
The complex trigonometric equation given in question 5(a)(i) could be differentiated directly as two terms, but it could also be simplified into a single Rsin(x+α) term first. Most students attempted the two-term differentiation, but the guidance column outlines the partial credit steps if the single trig term is found.

Mark scheme Question 5(a)(i) H240/2 2023
The guidance makes clear that there are follow on marks available in part (a)(ii) dependent upon the route taken.
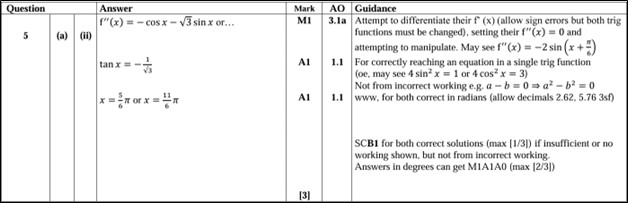
Mark scheme Question 5(a)(ii) H240/2 2023
Either trigonometric route is prone to mistakes with positive/negative signs and sine/cosine confusions. The DR instruction is there to remind students to set out their working clearly, so that the assessor can easily see which route has been attempted and mark accordingly. This is especially true in this part where evidence of using their result from part (i) will help secure the first M mark in part (ii).
Always set out written working neatly so that the examiner can award method marks for what you have attempted.
In summary
The DR statement highlights to students that the examiner will be looking as carefully at the working as they are looking at the final answer. Make sure each line of reasoning follows clearly from the previous, and don’t skip any steps of that logical process.
Use correct mathematical notation, equations should have an equals sign, integrations need limits and clear statement of the variables involved and take care where inequalities are strictly inequalities or if the ‘equals’ is included. Don’t just do the calculations, make it clear what the calculations represent.
Stay connected
Share your thoughts in the comment box below. Don’t forget to join us for our Teacher Network events.
If you have any queries, you can email us at maths@ocr.org.uk, call us on 01223 553998 or message us on X @OCR_Maths. You can also sign up to subject updates and receive information about resources and support.
If you are considering teaching any of our qualifications, use our online form to let us know, so that we can help you with more information.
About the author
Steven originally studied engineering before completing a PGCE in secondary mathematics. He has taught secondary maths in England and overseas. Steven joined OCR in 2014 and worked on the redevelopment of OCR’s FSMQ and the A Level Mathematics suite of qualifications. Steven normally drafts the weekly #OCRMathsPuzzle and then the rest of the team review, finalise and then publish each Friday. Away from the office he enjoys cooking and to travel.
Related blogs